The N-particle partition function for distinguishable particles

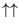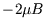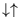Let's start with two spins. There are four states of the whole system, $\uparrow \uparrow$ with energy $-2\mu B$, $\uparrow \downarrow$ and $\downarrow\uparrow$, both with energy zero, and 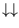$\downarrow\downarrow$ with energy 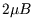$2\mu B$. Thus the two-particle partition function is

$$\begin{eqnarray*} Z_2\!\!\!&=&\!\!\!e^{ 2\mu B\beta}+e^0+e^0+e^{-2\mu B\beta}=e... ...\ \!\!\!&=&\!\!\!(e^{ \mu B\beta}+e^{-\mu B\beta})^2 =(Z_1)^2 \end{eqnarray*}$$

In general, for 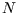$N$ particles, the energies range through 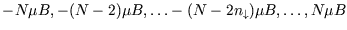$-N\mu B, -(N-2)\mu B,\ldots -(N-2n_\downarrow )\mu B,\ldots, N\mu B$ with there being 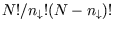$N!/n_\downarrow !(N-n_\downarrow )!$ separate states with 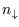$n_\downarrow$ down-spins. So

$$\begin{eqnarray*} Z_N\!\!\!&=&\!\!\!e^{-N\mu B\beta}+\ldots +{N!\over n_\downarr... ...\\ \!\!\!&=&\!\!\!(e^{-\mu B\beta}+e^{ \mu B\beta})^N =(Z_1)^N \end{eqnarray*}$$

The treatment for a system with more than two single-particle states is covered here.

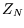There is a caveat, which can be ignored on first reading. The argument says that there are a number of different states with the same number of down spins. Since the spins are arranged on a lattice, this is correct; every spin can be distinguished from every other spin by its position. When we go on to consider a gas, however, this is no longer so, and the relation between $Z_1$ and $Z_N$ changes. The treatment for indistinguishable particles is here.