PC1672 Advanced dynamics

4.3 Tensors

A tensor, like a vector, is a mathematical object with a geometrical meaning. A tensor acts on a vector to produce a new vector which is linearly related to the old one but points in a different direction (in general). In some coordinate basis, the components of a tensor form a $3\times 3$ matrix. However the action of a tensor on a vector is independent of the basis we use to represent them and so its geometrical meaning is invariant.

An important physical example is the stress tensor $\underline{\underline{\bf T}}$ which relates the force transmitted across some surface in a solid to the (vector) area $\delta{\bf A}$ of that surface:

$\begin{displaymath}\delta{\bf F}=\underline{\underline{\bf T}}\cdot\delta{\bf A}\end{displaymath}$

Another example is provided by electric and magnetic fields which, in special relativity, form the components of a four-dimensional tensor. The metric tensor generalises Pythagoras's expression for the distance between two points to curved spaces. The four-dimensional version of this tensor plays a central role in general relativity. The action of a tensor $\underline{\underline{\bf T}}$ on a vector ${\bf u}$ will be denoted here by

$\begin{displaymath}{\bf v}=\underline{\underline{\bf T}}\cdot{\bf u}\end{displaymath}$

In component form, this is just matrix multiplication

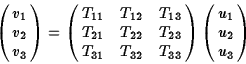
$\begin{displaymath}\pmatrix{v_1\cr v_2\cr v_3}=\pmatrix{T_{11}&T_{12}&T_{13}\cr ... ...{22}&T_{23}\cr T_{31}&T_{32}&T_{33}}\pmatrix{u_1\cr u_2\cr u_3}\end{displaymath}$

$\begin{displaymath}T_i=\sum_j T_{ij}u_j\end{displaymath}$

This is a linear operation and so it satisfies the distributive laws

$\begin{displaymath}\underline{\underline{\bf T}}\cdot({\bf u}+{\bf v}) =\underli... ...{\bf T}}\cdot{\bf u} +\underline{\underline{\bf T}}\cdot{\bf v}\end{displaymath}$

and

$\begin{displaymath}(\underline{\underline{\bf S}}+\underline{\underline{\bf T}})... ...{\bf S}}\cdot{\bf u}+\underline{\underline{\bf T}}\cdot {\bf u}\end{displaymath}$

(just like matrix multiplication).

The unit tensor $\underline{\underline{\bf 1}}$ leaves all vectors unchanged:

$\begin{displaymath}\underline{\underline{\bf 1}}\cdot{\bf u}={\bf u}\quad\hbox{\rm for all {\bf u}}\end{displaymath}$

Its components form the $3\times 3$ identity matrix

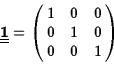
$\begin{displaymath}\underline{\underline{\bf 1}}=\pmatrix{1&0&0\cr 0&1&0\cr 0&0&1}\end{displaymath}$

They can also be expressed as

$\begin{displaymath}\left(\underline{\underline{\bf 1}}\right)_{ij}=\delta_{ij}\end{displaymath}$

where the Kronecker delta is defined by

$\begin{displaymath}\delta_{ij}=\left\{{1\quad \hbox{\rm if}\quad i=j\atop 0\quad \hbox{\rm if}\quad i\neq j}\right.\end{displaymath}$

You should be familiar with the scalar and vector product of two vectors. There is a third way to multiply two vectors: the dyadic product which yields a tensor. It is denoted ${\bf a}\otimes{\bf b}$ and has components

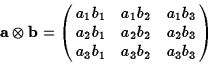
$\begin{displaymath}{\bf a}\otimes{\bf b}=\pmatrix{a_1b_1&a_1b_2&a_1b_3\cr a_2b_1&a_2b_2&a_2b_3\cr a_3b_1&a_3b_2&a_3b_3}\end{displaymath}$

This type of tensor is also known as a second-rank tensor, where the rank refers to the number of indices on its components. A scalar can be thought of as a zeroth-rank tensor and a vector as a first-rank tensor. More complicated kinds also exist. The crucial property of a tensor is that that its components should transform under a rotation of coordinate axes in such a way as to keep its geometrical or physical meaning invariant.

More on tensors: This type of tensor is also known as a second-rank tensor, where the rank refers to the number of indices on its components. A scalar can be thought of as a zeroth-rank tensor and a vector as a first-rank tensor. More complicated kinds also exist. The crucial property of a tensor is that that its components should transform under a rotation of coordinate axes in such a way as to keep its geometrical or physical meaning invariant.

Textbook references

K&K 7.6
F&C 9.1
B&O 2.4 pages 57-58, 6.7
M 12.2
M&T 11.2
B 10.10-10.12 for mathematical aspects of tensors
Feynman lectures, volume III, 31 for uses of tensors in physics

Mike Birse
21st July 2000