Home: PC 1672 home page |
Up: 3 Gravity |
Weekly plan |
Help: Guide to using this document |
Next: 3.5 Reduced mass |
Previous: 3.3 . . . potential energy |
The gravitational potential at a point ![]() is defined as the
potential energy of a unit mass at that point (
is defined as the
potential energy of a unit mass at that point (![]() ). It is related
to the gravitational field by
). It is related
to the gravitational field by
We can calculate the potential due to a general extended body by chopping
the body up into small elements which we can treat like point masses. The
total potential is then found by summing up (integrating) the potentials
of the elements. For a body of volume ![]() and density
and density
![]() we have
we have
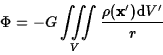
In the case of a thin spherical shell of matter with mass ![]() and radius
and radius ![]() ,
we find that the potential at distance
,
we find that the potential at distance ![]() from the centre is
from the centre is
A general spherical distribution of matter can be broken up into to concentric
spherical shells. Using the results above we see that only the matter inside
a radius ![]() contributes to the field at
contributes to the field at ![]() ,
and that its contribution looks
just like that of a point mass at the centre. In terms of the total mass
,
and that its contribution looks
just like that of a point mass at the centre. In terms of the total mass
![]() inside radius
inside radius ![]() ,
the field is
,
the field is
Gauss's law: The gravitational field satisfies
Gauss's
law in the form
Textbook references
Home: PC 1672 home page |
Up: 3 Gravity |
Weekly plan |
Help: Guide to using this document |
Next: 3.5 Reduced mass |
Previous: 3.3 . . . potential energy |
Mike Birse
17th May 2000