Hint 3

The pericentre distance is

$$r_1=a(1-\epsilon)=200\; {\rm km}$$

which corresponds to 190 km above the surface.

The period (which is equal to that for a circular orbit of radius 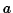$a$) is

$$T=2\pi\sqrt{a^3\over GM}=16\; {\rm days}$$

since the mass of the asteroid is 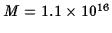$M=1.1\times 10^{16}$ kg.

The energy (also equal to that for a circular orbit of radius 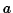$a$) is

$$E=-{GMm\over 2a}$$

Hence the energy must decrease by

$$\Delta E={GMm\over 2a}=570\; {\rm J}$$

since the energy of a parabolic orbit is zero.

The maximum speed occurs at pericentre where the energy can be written

$$E={1\over 2}mv_1^2-{GMm\over r_1}$$

Using the total energy above gives

$$v_1=\sqrt{GM\left({2\over r_1}-{1\over a}\right)}=2.3\;{\rm ms}^{-1}$$