PHYS20672 Summary 2

Conformal mappings

1. If $f=u+iv$ is analytic, contours of constant $u$ and $v$ intersect each other at right angles.
2. The mapping $w=f(z)$ preserves angles and, for sufficiently small regions, shapes. The mapping is called conformal.
3. Suppose we wish to find $\phi(x,y)$, the solution to Laplace's equation subject to certain boundary conditions, and that the mapping $Z=f(z)$ maps the boundaries to a new simpler geometry in which Laplace's equation can be solved, giving $\tilde\phi(X,Y)$. Then $\phi(x,y)=\tilde\phi(X(x,y),Y(x,y))$ will be the solution to Laplace's equation original problem.
4. If $\psi(x,y)$ is conjugate to $\phi(x,y)$, lines of constant $\psi(x,y)$ represent field lines, lines of heat flow or streamlines according to the physical interpretation of $\phi$. The analytic function $\phi+i\psi$ is called the "complex potential"

In the diagrams above the blue and red lines equipotentials and flow/field lines for a variety of problems.

Riley 18.7-9; Boas 14.9, 14.10; (Arfken 6.7)

Integration in the complex plane

1. The path (or contour) integral of a complex function is defined as $$\int_C f(z)\d z=\lim_{n\to\infty}\sum_{k=1}^{n} f(\xi_k)(z_k-z_{k-1}),$$ where $z_0$ and $z_n$ are the end-points of the contour, the other $z_k$ are points distributed in order along the contour, and the points $\xi_k$ are chosen so that $z_{k-1}< \xi_k < z_k$. Provided $f(z)$ is continuous and the limit is taken so that all $(z_k-z_{k-1})\to 0$, the limit is unique for a given path.

   

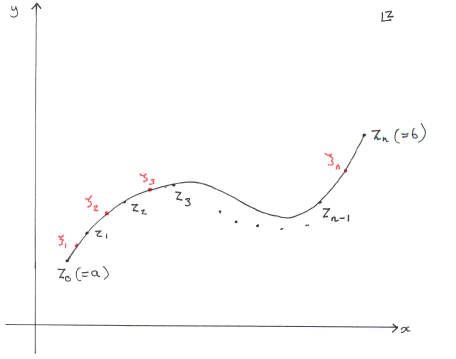
2. For real functions of $x$ only this corresponds to the usual integral, ie the area under the curve $f(x)$.
3. We have $$\int_C f(z)\d z=\int u\d\,x-v\d\,y+i\int v\d\,x+u\d\,y=\int(u,-v)\cdot\underline{\d\,l}+i\int(v,u)\cdot\underline{\d\,l}$$ where the integrals are real line integrals in the $xy$-plane.
4. Reversing the direction of the contour changes the sign of the integral.
5. For compound curves $$\int_{C_1+C_2} f(z)\d z=\int_{C_1} f(z)\d z+\int_{C_2} f(z)\d z\qquad\text{and}\qquad\int_{C_1-C_2} f(z)\d z=\int_{C_1} f(z)\d z-\int_{C_2} f(z)\d z$$.
6. The estimation lemma states $|\int_C f(z)\d z|\le ML$ where $L$ is the length of the curve and $M$ is the maximum value of $|f(z)|$ along the curve.
7. A Jordan curve is a closed non-self-intersecting curve. The integral along such a curve is denoted by $\oint$, and is taken in an anticlockwise direction.

Riley 18.10; (Boas 14.3); Arfken 6.3