The ﬁne structure of hydrogen

3.3 The ﬁne structure of hydrogen

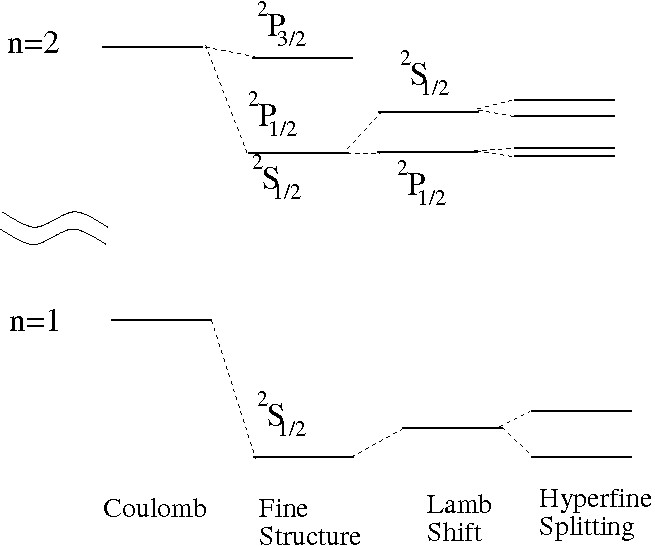
Although the Schrödinger equation with a Coulomb potential reproduces the Bohr model and gives an excellent approximation to the energy levels of hydrogen, the true spectrum was known to be more complicated right from the start. The small deviations are termed “ﬁne structure” and they are of order $1 0^{- 4}$ compared with the ground-state energy (though the equivalent terms for many-electron atoms can be sizable). Hence perturbation theory is an excellent framework in which to consider them.

There are two eﬀects to be considered. One arises from the use of the non-relativistic expression $p^{2} ∕ 2 m$ for the kinetic energy, which is only the ﬁrst term in an expansion of $\sqrt{{(m c^{2})}^{2} + {(p c)}^{2}} - m c^{2}$ . The ﬁrst correction term is $- p^{4} ∕ (8 m^{3} c^{2})$ , and its matrix elements are most easily calculated using the trick of writing it as $- 1 ∕ (2 m c^{2}) {({\hat{H}}^{(0)} - V_{C} (r))}^{2}$ , where ${\hat{H}}^{(0)}$ is the usual Hamiltonian with a Coulomb potential. Now in principle we need to be careful here, because ${\hat{H}}^{(0)}$ is highly degenerate (energies depend only on $n$ and not on $l$ or $m$ ). However we have $⟨ n l^{'} m^{'} | {({\hat{H}}^{(0)} - V_{C} (r))}^{2} | n l m ⟩ = ⟨ n l^{'} m^{'} | {(E_{n}^{(0)} - V_{C} (r))}^{2} | n l m ⟩$ , and since in this form the operator is spherically symmetric, it can’t link states of diﬀerent $l$ or $m$ . So the basis ${| n l m ⟩}$ already diagonalises ${\hat{H}}^{(1)}$ in each subspace of states with the same $n$ , and we have no extra work to do here. (We are omitting the superscript $^{(0)}$ on the hydrogenic states, here and below.)

The ﬁnal result for the kinetic energy eﬀect is

⟨ n l m | {\hat{H}}_{K E}^{(1)} | n l m ⟩ = - \frac{α^{2} |E_{n}^{(0)}|}{n} (\frac{2}{2 l + 1} - \frac{3}{4 n})

In calculating this the expressions $E_{n}^{(0)} = \frac{1}{2 n^{2}} α^{2} m c^{2}$ and $a_{0} = ℏ ∕ (m c α)$ are useful. Tricks for doing the the radial integrals are explained in Shankar qu. 17.3.4; they are tabulated in section A.3. Details of the algebra for this and the following calculation are given here.

The second correction is the spin-orbit interaction:

{\hat{H}}_{SO}^{(1)} = \frac{1}{2 m^{2} c^{2} r} \frac{d V_{C}}{d r} \hat{L} \cdot \hat{S}

In this expression $\hat{L}$ and $\hat{S}$ are the vector operators for orbital and spin angular momentum respectively. The usual (somewhat hand-waving) derivation talks of the electron seeing a magnetic ﬁeld from the proton which appears to orbit it; the magnetic moment of the electron then prefers to be aligned with this ﬁeld. This gives an expression which is too large by a factor of 2; an exact derivation requires the Dirac equation.

This time we will run into trouble with the degeneracy of ${\hat{H}}^{(0)}$ unless we do some work ﬁrst. The usual trick of writing $2 \hat{L} \cdot \hat{S} = {\hat{J}}^{2} - {\hat{L}}^{2} - {\hat{S}}^{2}$ where $\hat{J} = \hat{L} + \hat{S}$ tells us that rather than working with eigenstates of ${\hat{L}}^{2}, {\hat{L}}_{z}, {\hat{S}}^{2}$ and $Ŝ_{z}$ , which would be the basis we’d get with the minimal direct product of the spatial state $| n l m_{l} ⟩$ and a spinor $| s m_{s} ⟩$ , we want to use eigenstates of ${\hat{L}}^{2}, {\hat{S}}^{2} {\hat{J}}^{2}$ and $Ĵ_{z}$ , $| n l j m_{j} ⟩$ , instead. (Since $S = \frac{1}{2}$ for an electron we suppress it in the labelling of the state.) An example of such a state is $| n 1 \frac{1}{2} \frac{1}{2} ⟩ = \sqrt{\frac{2}{3}} | n 11 ⟩ \otimes | \frac{1}{2} - \frac{1}{2} ⟩ - \sqrt{\frac{1}{3}} | n 10 ⟩ \otimes | \frac{1}{2} \frac{1}{2} ⟩$ .

Then

⟨ n l j m_{j} | {\hat{H}}_{S O}^{(1)} | n l j m_{j} ⟩ = \frac{α^{2} |E_{n}^{(0)}|}{n} (\frac{2}{2 l + 1} - \frac{2}{2 j + 1})

(This expression is only correct for $l \neq 0$ . However there is another separate eﬀect, the Darwin term, which only aﬀects $s$ -waves and whose expectation value is just the same as above (with $l = 0$ and $j = \frac{1}{2}$ ), so we can use this for all $l$ . The Darwin term can only be understood in the context of the Dirac equation.)

So ﬁnally

E_{n j}^{(1)} = \frac{α^{2} |E_{n}^{(0)}|}{n} (\frac{3}{4 n} - \frac{2}{2 j + 1}) .

The degeneracy of all states with the same $n$ has been broken. States of $l = j \pm \frac{1}{2}$ are still degenerate, a result that persists to all orders in the Dirac equation (where in any case orbital angular momentum is no longer a good quantum number.) So the eight $n = 2$ states are split by $4.5 \times 1 0^{- 5}$ eV, with the $^{2} p_{3 ∕ 2}$ state lying higher that the degerate $^{2} p_{1 ∕ 2}$ and $^{2} s_{1 ∕ 2}$ states.

Two other eﬀects should be mentioned here. One is the hyperﬁne splitting. The proton has a magnetic moment, and the energy of the atom depends on whether the electon spin is aligned with it or not— more precisely, whether the total spin of the electon and proton is $0$ or $1$ . The anti-aligned case has lower energy (since the charges are opposite), and the splitting for the $1 s$ state is $5.9 \times 1 0^{- 6}$ eV. (It is around a factor of 10 smaller for any of the $n = 2$ states.) Transitions between the two hyperﬁne states of $1 s$ hydrogen give rise to the 21 cm microwave radiation which is a signal of cold hydrogen gas in the galaxy and beyond.

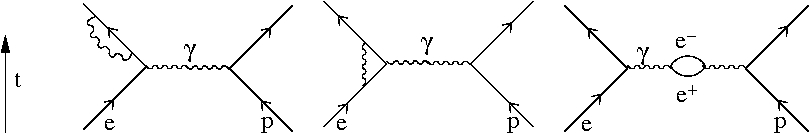
The ﬁnal eﬀect is called the Lamb shift. It cannot be accounted for in quantum mechanics, but only in quantum ﬁeld theory.

The diagrams above show corrections to the simple Coulomb force which would be represented by the exchange of a single photon between the proton and the electron. The most notable eﬀect on the spectrum of hydrogen is to lift the remaining degeneracy between the $^{2} p_{1 ∕ 2}$ and $^{2} s_{1 ∕ 2}$ states, so that the latter is higher by $4.4 \times 1 0^{- 6}$ eV.

Below the various corrections to the energy levels of hydrogen are shown schematically. The gap between the $n = 1$ and $n = 2$ shells is supressed, and the Lamb and hyperﬁne shifts are exaggerated in comparison with the ﬁne-structure. The eﬀect of the last two on the $^{2} p_{3 ∕ 2}$ level is not shown.

Gasiorowicz ch 12.1,2,4
Mandl ch 7.4
Shankar ch 17.3
Townsend ch 11.6,7

[next] [prev] [up] [top]