Home: PC 1672 home page |
Up: 1 Relativity |
Weekly plan |
Help: Guide to using this document |
Next: 1.3 Proper time |
Previous: 1.1 Symmetry in physics |
A central feature of
Einstein's
Special Relativity is that the speed of light is the same in all
inertial frames. As a result we have to give up
on the idea of a universal time. If an event occurs at position
![]() and time
and time ![]() as measured by an observer in frame
O then for an observer in a frame O' moving with velocity
as measured by an observer in frame
O then for an observer in a frame O' moving with velocity
![]() in the
in the ![]() -direction it occurs at
-direction it occurs at
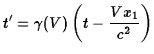 |
This relation between the space and time coordinates of an event in the two
frames is the
Lorentz
transformation for observers with relative
velocity ![]() in the
in the ![]() -direction. For a Lorentz boost to a frame moving
in a general direction, the transformation has
a similar form, mixing the coordinate in the boost direction with the time,
and leaving the coordinates in perpendicular directions unchanged.
-direction. For a Lorentz boost to a frame moving
in a general direction, the transformation has
a similar form, mixing the coordinate in the boost direction with the time,
and leaving the coordinates in perpendicular directions unchanged.
The Lorentz transformation leaves unchanged the quantity
If we introduce the coordinate
![]() then this invariant can be
rewritten as
then this invariant can be
rewritten as
We can think of this vector as the position vector of
the event in a four-dimensional space-time continuum (often called
Minkowski
space). In the same way that a real rotation leaves the length of a vector
invariant, a Lorentz transformation does not change ![]() .
To distinguish them from ordinary
three-dimensional quantities, we shall refer to these objects as
4-vectors and 4-scalars.
.
To distinguish them from ordinary
three-dimensional quantities, we shall refer to these objects as
4-vectors and 4-scalars.
Textbook references
Home: PC 1672 home page |
Up: 1 Relativity |
Weekly plan |
Help: Guide to using this document |
Next: 1.3 Proper time |
Previous: 1.1 Symmetry in physics |
Mike Birse
17th May 2000